Abstract
When drug products contact plastic manufacturing components, packaging systems, and/or delivery devices, leachables from the plastics can accumulate in the drug product, potentially affecting its key quality attributes. Given practical issues associated with screening drug products for leachables, potential leachables are frequently surfaced as extractables revealed in extraction studies. To facilitate extractables discovery and identification and to shorten extraction times, extraction studies can be exaggerated and/or accelerated. One means of exaggerating an extraction is to increase the test article's extracted surface area to extraction solution volume ratio (SA/V), as it is generally accepted that an extractable's concentration in an extract is proportional to SA/V in a 1 to 1 manner. However, as the relationship between an extractable's concentration and SA/V depends on the extractable's plastic/solvent partition coefficient (kp/l), the effect of SA/V on the extractable's concentrations can be either under- or over-estimated if a 1 to 1 proportion is used. This article presents the theoretical relationship between SA/V, concentration, and kp/l; illustrates theory with a case study; and suggests proper exaggeration strategies.
LAY ABSTRACT: When drug products are manufactured, stored, or delivered in systems that contain plastics, substances can be leached from the plastics and remain in the drug product, where they might affect the product's key quality attributes. To discover and identify these leached substances, the plastics are extracted under laboratory conditions and the extracts are appropriately tested. To facilitate this process, extracts may be generated under laboratory conditions that exaggerate or accelerate the drug product's clinical conditions of manufacturing or use. The proper use of the ratio of the extracted item's surface area to the volume of the extracting solution as an exaggeration parameter is discussed in this paper.
Introduction
During their production, storage, and clinical use, drug products and their production precursors likely come in contact with plastic manufacturing components, packaging systems, and medical devices used in their administration. During contact, substances present in the plastic may leach into the drug product or production precursor, thereby potentially affecting key quality attributes of the drug product (for example, stability, potency, safe use, etc.).
As screening drug products for leachables can be analytically challenging, potential leachables are often established by performing extraction studies on the components, systems, or devices. As extractables tend to be (a) present in plastics in relatively small quantities and/or (b) leached from plastics in small quantities, the concentration of extractables in extracts can be low, complicating extractables' discovery, identification, and quantitation. Thus, extractions that exaggerate the clinical conditions of contact may be used to produce a more concentrated extract (that is, an extract that has higher levels of a greater number of extractables). Furthermore, as contact between the drug product (or its precursors) may occur over longer periods of time (for example, packaged drug products may have shelf-lives that are measured in years), extraction studies that use actual clinical conditions of contact may require impractically long durations. Thus, extractions that accelerate leaching by exaggerating the conditions of contact (for example, temperature or extracted surface area) may be employed to make extraction times more manageable.
Although several experimental factors may be modified (versus clinical use) to achieve exaggeration or acceleration, this article specifically considers the ratio of the surface area of a test sample contacted by an extracting solution to the volume of the extraction solution (SA/V). It is generally accepted that as SA/V increases, so too will the concentration of an extractable in the extracting solution. For example, when a drug product is packaged in containers that can vary in size, it is typically the case that the smallest available size (fill volume) is tested, as the package's surface area to fill solution volume ratio (SA/V) is largest for the smallest available size. Another example could involve a package that holds 50 mL of drug product and that has an access site that includes a septum. In an exaggerated extractables study one might extract five septa per 50 mL of extraction solvent in the belief that so doing will produce a more concentrated extract, thus facilitating the discovery and identification of extractables.
Moreover, when the consequence of changing the SA/V to the extractables profile is calculated, an adjustment factor is used and is frequently based, on the assumption that there is a one-to-one relationship between SA/V and the extractable's concentration in the extract; for example, doubling SA/V will double the extractable's concentration in the extract.
In some situations, the exaggeration is the basis of a claim that the extraction conditions used reflect a worst-case extraction. In other situations, exaggeration factors are used to forecast what extractables levels would be under the conditions of use when the conditions of the extraction differ from the conditions of use. In the case of the access site on a container noted previously, for example, one might divide the concentration of an extractable measured in the extract by the 5-fold “exaggeration factor” to estimate the levels of the extractable (as a leachable) in the drug product based on an underlying assumption that the level of extractables in an extracting solution (or leachables in a drug product) will increase in direct proportion to an increasing SA/V. Although such an assumption is intuitively attractive, it is not based on sound science and in fact is contradicted when the extraction process is considered on the basis of scientific first principles. Thus, indiscriminate use of this assumption can produce irrelevant, inaccurate, and inappropriate extrapolations.
The purpose of this article is to establish and justify appropriate practices related to exaggerating extraction conditions used in extractables studies, specifically related to SA/V and specifically focusing on those circumstances when equilibrium is achieved between the extracted test article and the extracting medium.
Discussion
General
A test article's SA/V can affect both the thermodynamics and kinetics involved with extraction or leaching. Thermodynamically, the greater the SA/V, the greater the total pool of an extractable or leachable. Kinetically, an increased SA/V will increase the speed of extraction or leaching, not necessarily by increasing the extractable's diffusion rate through the plastics but by increasing the rate at which the extractable leaves the plastic and enters the solution.
If the extraction or leaching conditions (e.g., duration and temperature) are such that equilibrium is attained between the test article being extracted and the extracting solution, then the kinetic effect is irrelevant and only the thermodynamic aspects are important to consider. This is the circumstance that is addressed by this article.
Theoretical
The equilibrium partitioning of a substance between a plastic phase (such as a package, a manufacturing component, or a medical device) and a liquid solution phase (such as the drug solution or an extracting solution) is described by a plastic/liquid partition coefficient, kp/l, as follows:
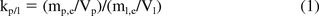where m = the mass of a substance in a particular phase, V is the volume of either phase and the subscripts p, l, and e refer to the plastic phase, the liquid phase, and equilibrium, respectively.
Equation 1 can be re-written to express the relationship between the concentration of a substance in the liquid phase at equilibrium (Cl,e) and the various other relevant parameters as shown in eq 2 (obtained from reference 1):
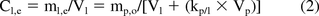where the subscript o refers to the initial condition prior to contact.
Now because the volume of an extracted portion of a plastic is the product of the plastic's surface area (SAp) and its thickness (tp), Vp = SAp/tp, eq 2 can be re-arranged to produce a relationship that expresses the substance's solution level at equilibrium as a function of the extracted material's surface area and the extraction solution's volume:
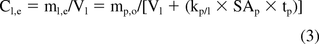As noted from eq 3, a substance's plastic/solution partition coefficient (kp/l) will mediate the effect that changing SA/V has on the substance's equilibrium concentration in solution (Cl,e). It is also noted that eq 3 does not fully consider certain complicating factors such as irregularly shaped test articles and the use of penetrating extraction solvents; nevertheless, the equation provides some insight into SA/V effects even in these more complicated circumstances.
To examine this effect more quantitatively, the following example is considered:
mp,o = 10 mg/cm2,
Vl = 100 mL = 100 cm3,
tp = 1 cm, and
kp/l takes values ranging from 0.1 (substance which partitions favorably into the extracting solution) to 1000 (substance which partitions favorably into the extracted plastic).
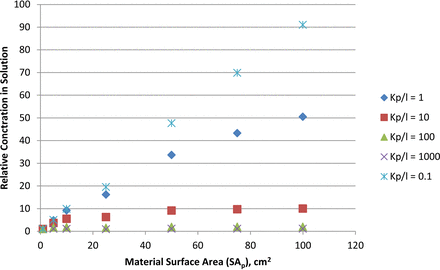
Under these conditions, the effect of increasing the surface area of the plastic (SAp) on the equilibrium concentration of the substance in the solution (Cl,e) is shown in Figure 1. Consistent with eq 3, the effect of surface area is greatly reduced as the substance's kp/l increases. For a substance that is highly soluble in the solution, an increase in material surface area produces nearly a proportional increase in the concentration of the substance in the solution. For example, when the surface area is increased by a factor of 100 for a substance with a kp/l of 0.1, the increase in the substance's concentration in solution is also nearly a factor of 100. On the other hand, for a substance that is poorly soluble in the solution (kp/l = 100), a 100-fold increase in surface area produces barely a doubling of the substance's concentration in solution.
Relationship between the material surface area and the relative concentration of an extractable in an extracting solution at a constant extracting solution volume. The relationship is shown for extractables with kp/l ranging from 0.1 (extractable is highly soluble in the solution) to 1000 (extractable is poorly soluble in the solution).
Two scenarios were considered in the Introduction in terms of the use of exaggerated SA/Vs. The first scenario addressed the situation where a family of products, differing in size, were deemed to be represented by that one product that had the highest SA/V. For a package, the highest SA/V is attained by the smallest sized package in the family, as it is usually the case that the size of the package does not increase in direct proportion to its fill volume. The rationale for choosing the size with the largest SA/V was that this would be the product size that had the highest concentration of substances in solution and thus which was the worst case in terms of substance concentration. The analysis shown in Figure 1 confirms this rationale, as it is always the case that the substance's concentration in solution is highest at the highest SA/V.
The second scenario considered in the Introduction involved the use an exaggeration factor to extrapolate the concentration of an extractable in the extracting solution obtained at one value of SA/V to the concentration in of the same extractable in an extracting solution at another value of SA/V. In this situation, the exaggeration factor would be the magnitude of the difference in the SA/Vs. More specifically, if SA/V in case 2 was twice the SA/V in case 1, then the solution concentration of a substance in case 2 would be twice the solution concentration in case 1.
Figure 1 clearly shows that this intuitive approach is not always proper and correct. While a 100-fold increase in SA/V produces a nearly 100-fold increase in the solution concentration for a substance that is highly soluble in the solution (kp/l = 0.1), the increase is much less even for relatively soluble substances (for example, the increase is roughly 50-fold when the kp/l is 1). For poorly soluble substances (kp/l = 1000), a 100-fold increase in SA/V produces less than a 10% increase in the substance's solution concentration.
Proper Exaggeration Factors
It is often the case that not all extractables are known at the start of an extraction study as the purpose of the extraction study is to discover, identify and quantify extractables. In this circumstance, it is clear that the kp/l values for many extractables will also be unknown and thus it will not be possible to design an exaggerated extraction with an appropriate and justified exaggeration factor. Thus while it is clear that 100-fold SA/V extrapolations are not proper or viable in many cases (as such a large exaggeration would surely produce exaggeration factors that were significantly less than 100), it may be the case that smaller extrapolations would be more generally acceptable. Table I addresses this possibility by collating the concentration exaggeration factors when SA/V is changed by a factor of 5 or less.
Relative Concentrations in Solution at Various Surface Area to Volume Ratios
One notes from Table I that when the surface area is increased from 1 to 3 (a potential exaggeration factor of 3), even in the case of a poorly soluble compound (kp/l = 100), the actual exaggeration factor is close to the potential factor (1.5 versus 3, or approximately 50%). However, at a surface area exaggeration factor of 5, the actual exaggeration factor for a substance with kp/l = 100 is only 33% of its potential value. Thus in order to provide an exaggeration that can effectively be linked back to the actual clinical conditions of contact, the exaggeration factor must be limited to a certain range; typically, an exaggeration of 5 or less will produce extractables concentrations that are roughly proportional to the exaggeration factor.
Case Study
One of the examples previously considered was the case of a drug product that is commercially available in multiple package sizes (e.g., multiple fill volumes), as can be the case for certain drug products used as parenteral injections. The measurement of targeted leachables over storage time across multiple package sizes for one or more parenteral solutions of similar composition produces a set of data relevant to a consideration of the effect of SA/V on the leachable's concentration in the drug product.
The test articles for this case study were three different systems that are used to package aqueous parenteral injections such as sterile water, normal saline, and dextrose injections. Filled packages of multiple sizes (25–3000 mL) were treated in a manner appropriate for such products, which means that the filled units were terminally sterilized (autoclaved) and then stored for a period of time reflecting multiple year, ambient temperature shelf-lives. Over the course of such storage, the fill solutions were sampled and the samples tested for their levels of targeted leachables. Leachables were targeted based on their known association with the three container systems studied (see references 3⇓–5) and included di-(2-ethylhexyl) phthalate (DEHP) and Zn from a plasticized poly(vinyl chloride) package, caprolactam from a plastic package whose materials of construction included Nylon 6, and a class of compounds termed cyclic esters from a laminated plastic structure that included polyurethane-based tie layers. Validated analytical methods for quantifying the targeted leachables included inductively coupled plasma atomic emission spectroscopy (ICP-AES) for Zn, liquid chromatography with UV detection (LC/UV) for DEHP, liquid chromatography with mass spectrometric detection (LC/MS) for caprolactam, and gas chromatography with flame ionization detection (GC/FID) for the cyclic esters.
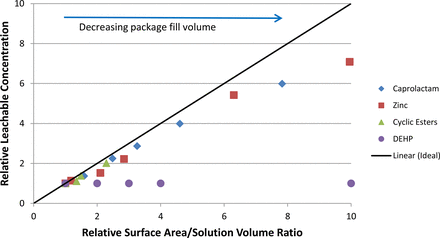
Figure 2 illustrates the effect of package size (SA/V) on the equilibrium concentration of the targeted leachables, where the equilibrium concentration represents the plateau concentration that was achieved during post-autoclave storage. In this figure, both the measured target concentration and SA/V are normalized to the value for the largest package (smallest SA/V and smallest extractable's concentration). The straight line shown on the graph is the “ideal” line that illustrates the case where concentration and SA/V are related in a 1-to-1 manner (for example, a 2-fold change in SA/V results in a 2-fold change in the leachable's concentration).
Normalized plot showing the experimental effect of a package's surface area to solution volume ratio (SA/V) on the equilibrium concentration of leachables in the contained solution. As the package size (fill volume) decreases, its SA/V increases, resulting in an increased extractable concentration in the contained solution. Concentrations and SA/V ratios have been normalized to the corresponding values for the largest package.
The divergence of the experimental data from the ideal line is clear for all the targeted leachables, consistent with the previous theoretical analysis. For those targeted leachables with a small kp/l (see Table II—these include caprolactam and two cyclic esters), the divergence is relatively small, as would be anticipated from eq 3. Alternatively, the high kp/l target, DEHP, exhibits almost no difference in leached concentration as a function of SA/V, also as anticipated from eq 3. The significance of the divergence is illustrated in the following situation. The equilibrium concentration of a targeted leachable has been measured in the largest sized package and the investigator seeks to extrapolate that outcome to a smaller package with a 5 times larger SA/V ratio. Were an idealized situation to be encountered, the investigator would project the leachable's concentration in the smaller package to be 5 times the level in the larger package. As shown in Figure 2, however, the actual “extrapolation factor” for the leachables with low partition coefficients would be closer to a factor of 4, yielding a lower concentration estimate. For certain leachables and in certain product use situations, the difference between a 4× and 5× concentration of a leachable in the packaged solution could be the difference between the leachable being above or below its safety-driven accumulation threshold.
Frequently Encountered Extractables and Their ko/w and kp/l Values
The over-exaggeration is far worse for a higher partition coefficient leachable such as DEHP. As was the case for the other leachables, the idealized extrapolation would indicate that the DEHP level in the smaller package should be 5 times that measured in the larger package. In fact the experimental data shows that the concentrations of DEHP in the two packages are essentially equal, as dictated by DEHP's limited aqueous solubility.
Thus extrapolating large package data to smaller packages based on the “idealized” circumstance over-estimates the leachable's concentration and over-estimates its safety risk. The higher the leachable's polymer/solution partition coefficient kp/l, the larger the over-estimation.
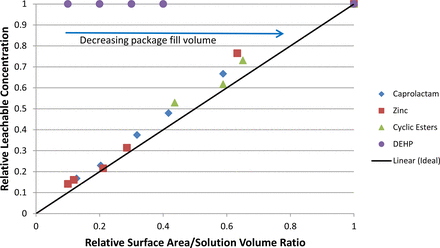
A more typical use of exaggeration factors is extrapolating the higher concentrations measured in the smallest package to lower concentrations relevant to larger packages. To illustrate this situation, Figure 3 is once again constructed from the experimental data, only this time the normalization is performed using the smallest package as the point of reference.
Normalized plot showing the experimental effect of a package's surface area to solution volume ratio (SV/A) on the equilibrium concentration of leachables in the contained solution. As the package's size (fill volume) decreases, its surface area to solution volume increases, resulting in an increased extractable concentration in the contained solution. Concentrations and SA/V ratios have been normalized to the corresponding values for the smallest package.
As was the case in Figure 2, Figure 3 shows a divergence between the experimental behavior and the idealized outcome. The significance of the divergence is illustrated in the following situation. The equilibrium concentration of a targeted leachable has been measured in the smallest sized package and the investigator seeks to extrapolate that outcome to a larger package that has a 5 times smaller SA/V ratio. Were the “idealized” situation to be encountered, the investigator would project the leachable's concentration in the larger package to be one-fifth its level in the smaller package. As shown in Figure 3, however, the actual “extrapolation factor” for the leachables with low partition coefficients would be closer to a factor of one-fourth, yielding a higher concentration estimate.
The under-exaggeration is far worse for a higher partition coefficient extractable such as DEHP. As was the case for the other leachables, the ideal extrapolation would indicate that the DEHP level in the smaller package should be one-fifth that measured in the larger package. In fact the experimental data shows that the concentrations of DEHP in the two packages are essentially equal.
Thus extrapolating small package data to larger packages based on the “ideal” circumstance under-estimates the leachable's concentration and under-estimates its safety risk. The higher the leachable's polymer/solution partition coefficient kp/l, the larger the under-estimation.
Additional Considerations
Cause and Effect?
To this point in this document, the phenomenon that is being investigated has been expressed in terms of a test article's SA/V. So doing implies a cause-and-effect relationship; that is, that it is the changed SA/V that causes the change in the extractable's concentration in the extract. However, in most situations, the increase in SA/V is also reflected in an increase in the mass of material extracted per volume of extracting solution, and it may be the case that it is the change in extracted mass, and not the change in surface area, that drives the change in the extractable's concentration. For example, the surface area and the material mass are proportionally related for a container whose composition and thickness is constant over the range of sizes.
Obtaining Polymer/Solution Partition Coefficients, kp/l, for Extractables
A polymer/solution partition coefficient (kp/l) is equivalent to a solvent/water partition coefficient (ks/w) in terms of its mathematical derivation and its application. If one could identify an organic solvent that behaved in the same manner as a particular polymer, then a substance's polymer/solution and solvent/water partition coefficients would be directly proportional.
Octanol/water partition coefficients (ko/w) are commonly available for a large number of organic substances (including extractables), thus making ko/w an excellent starting point for determining kp/l values. While octanol is not an exact model solvent for polymers used in pharmaceutical applications, relationships between kp/l and ko/w have been documented in the literature, for example, references 6 and 7. Such relationships for three types of polymers are as follows:
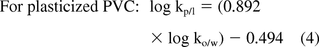
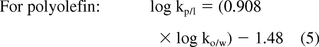
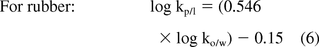Although these correlations are neither exact for a given polymer and certainly not applicable to all polymers of a given class, they are sufficiently applicable in a general sense that they can be used to estimate kp/l values for the purpose of designing extractables or leachables studies in terms of SV/A.
Table II lists ko/w values for a number of more commonly encountered extractables and provides kp/l values derived from the equations provided above.
Conclusions
Given the theoretical discussion provided previously and the results of the case study, the following conclusions are drawn:
Extractables concentrations can effectively be extrapolated as a function of SA/V so long as the exaggeration factor is no more than three.
Exaggeration factors larger than three can be justified on a case-by-case basis if the kp/l of the extractable can be established to be low (kp/l < 5).
Exaggeration factors larger than 10 should rarely be used and generally only in those circumstances where the kp/l values for all extractables are less than 1. Use of exaggeration factors greater than 10 would require justification on a case-by-case basis.
If one proportionally extrapolates concentrations from a lower SA/V to a higher SA/V, the extrapolated concentration will always be greater than or equal to the actual concentration (making the extrapolated concentration a worst case that will overestimate the actual case). The larger a substance's kp/l, the greater the relative difference between the extrapolated and actual concentrations.
If one proportionally extrapolates concentrations from a higher SA/V to a lower SA/V, the extrapolated concentration will not be greater than the actual concentration (and thus the extrapolated concentration will not be a worst case and will underestimate the actual case). The larger a substance's kp/l, the greater the relative difference between the extrapolated and actual concentrations.
As a corollary to point 5, an extractable's concentration measured at a higher SA/V ratio will always be equal to or greater than the extractable's concentration that would be measured at a lower SA/V ratio, making the higher SA/V a worse case for all lower SA/V ratios.
Conflict of Interest Declaration
The authors declare that they have no competing interests.
- © PDA, Inc. 2017
